next curve previous curve 2D curves 3D curves surfaces fractals polyhedra

CURVE WITH CONSTANT REACTION, L'HOSPITAL QUINTIC


The force applied by the marble on the support is constant


Problem of the "curva aequabilis pressionis" posed by Jean Bernoulli in 1695, solved by L'Hospital in 1700.
Other names: curve with constant pressure, "loop the loop" curve

 
Cartesian parametrization:  where .
The kinematic equation of motion is given by: .
Curvilinear abscissa and radius of curvature: .

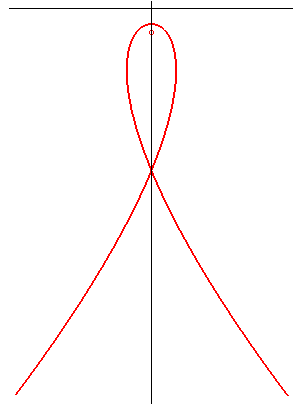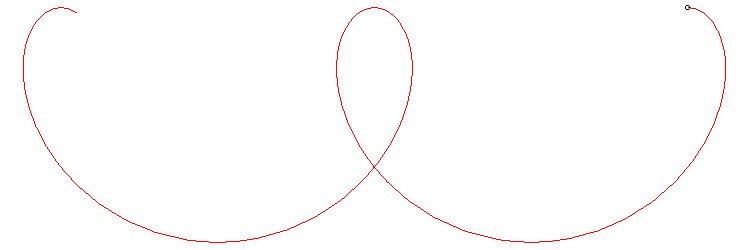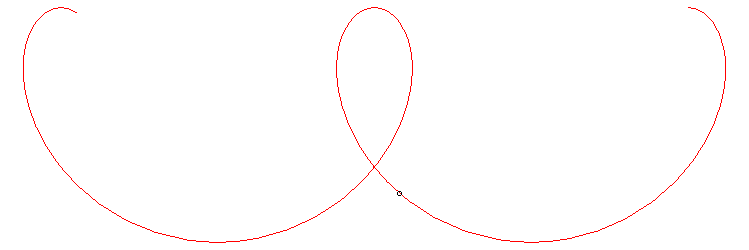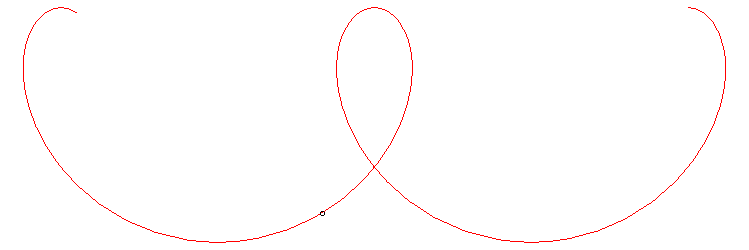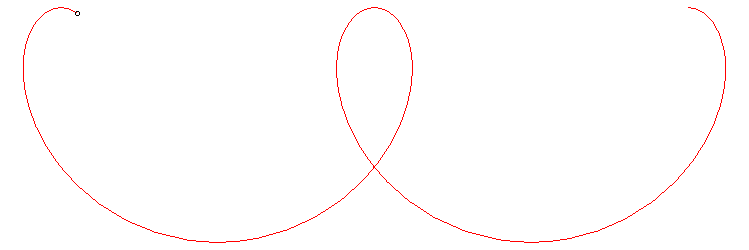
For k = 1 (case of the L'Hospital quintic) :
Cartesian parametrization:  ().
Cartesian equation:  or .
Polynomial quintic.
Vertex (0, a/4); isolated point ; 
double point .
Curvilinear abscissa: .
Radius of curvature: .
Parametrization of the evolute: .

A curve with constant reaction is a curve such that if a particle descends along it by the pull of gravity (the gravitational field is supposed to be uniform), then the reaction of the curve on the particle has a constant intensity; conversely, the force applied by the particle on the curve has a constant intensity.
 
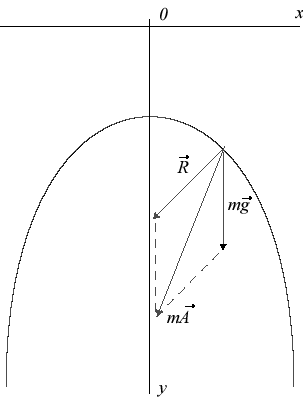With Oy as the descending vertical, Newton's law, projected on the normal, can be written: , where R is the intensity of the reaction force of the support, supposed to be constant (see the notations). The conservation of energy can be written: , with . Writing the ratio of the reaction and the weight of the particle , we get the differential equation of the curve: .

Solving it (use ) gives the parametrization above.
Be careful, this curve has constant reaction for only one value of the speed at the vertex: .

 
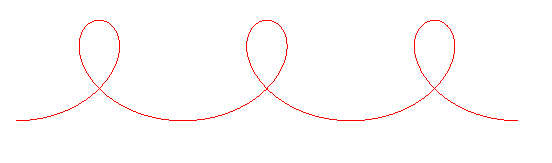cas réaction > poids
In the case where k > 1, the curve is transcendental, ( can be integrated thanks to inverse trig functions); the curve is periodic.
see the figure in the violet box
 In the case where k = 1, the curve is algebraic.
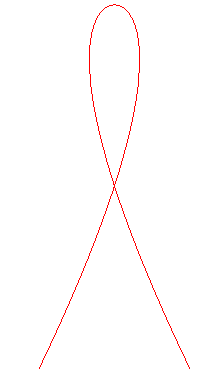cas réaction < poids
In the case where k < 1, the curve is transcendental again ( can be integrated thanks to inverse hyperbolic functions).

The evolute of such a curve is the solution of the following problem (also posed by Jean Bernoulli): determining a curve on which to wind the wire of a pendulum so that the tension of the wire of this pendulum remains constant.
 
Pendulum with constant tension; in red the curve with constant reaction, in blue its evolute.

For other curves of motion of a massive point in a gravitational field under certain conditions, see isochronous, brachistochrone and tautochronous.

Is this double loop-the-loop in Adventureland a curve with constant reaction?


next curve previous curve 2D curves 3D curves surfaces fractals polyhedra

© Robert FERRÉOL 2017